A Generic Quantum Wielandt’s Inequality

Republished By Plato

Followers: 0

Yifan Jia^1,2 and Angela Capel³

¹Department of Mathematics, Technische Universität München, Germany
²Munich Center for Quantum Science and Technology (MCQST), Germany
³Fachbereich Mathematik, Universität Tübingen, Germany

Find this paper interesting or want to discuss? Scite or leave a comment on SciRate.

Abstract

Quantum Wielandt’s inequality gives an optimal upper bound on the minimal length $k$ such that length-$k$ products of elements in a generating system span $M_n(mathbb{C})$. It is conjectured that $k$ should be of order $mathcal{O}(n^2)$ in general. In this paper, we give an overview of how the question has been studied in the literature so far and its relation to a classical question in linear algebra, namely the length of the algebra $M_n(mathbb{C})$. We provide a generic version of quantum Wielandt’s inequality, which gives the optimal length with probability one. More specifically, we prove based on [KS16] that $k$ generically is of order $Theta(log n)$, as opposed to the general case, in which the best bound to date is $mathcal O(n^2 log n)$. Our result implies a new bound on the primitivity index of a random quantum channel. Furthermore, we shed new light on a long-standing open problem for Projected Entangled Pair State, by concluding that almost any translation-invariant PEPS (in particular, Matrix Product State) with periodic boundary conditions on a grid with side length of order $Omega( log n )$ is the unique ground state of a local Hamiltonian. We observe similar characteristics for matrix Lie algebras and provide numerical results for random Lie-generating systems.

A generic quantum Wielandt’s inequality PlatoBlockchain Data Intelligence. Vertical Search. Ai.

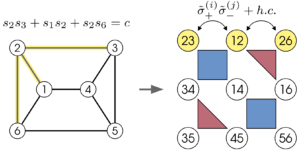
Featured image: Sketch of the proof for Theorem 1, a generic quantum Wielandt’s inequality.

► BibTeX data

@article{Jia2024genericquantum, doi = {10.22331/q-2024-05-02-1331}, url = {https://doi.org/10.22331/q-2024-05-02-1331}, title = {A generic quantum {W}ielandt’s inequality}, author = {Jia, Yifan and Capel, Angela}, journal = {{Quantum}}, issn = {2521-327X}, publisher = {{Verein zur F{“{o}}rderung des Open Access Publizierens in den Quantenwissenschaften}}, volume = {8}, pages = {1331}, month = may, year = {2024} }

► References

[1] I. Klep and Š. Špenko. “Sweeping words and the length of a generic vector subspace of mn(f)”. Journal of Combinatorial Theory, Series A 143, 56–65 (2016).
https://doi.org/10.1016/j.jcta.2016.05.003

[2] M. B. Hastings. “Solving gapped hamiltonians locally”. Phys. Rev. B 73, 085115 (2006).
https://doi.org/10.1103/PhysRevB.73.085115

[3] D. Perez-Garcia, F. Verstraete, M. M. Wolf, and J. I. Cirac. “Matrix product state representations”. Quantum Info. Comput. 7, 401–430 (2007).
https://doi.org/10.48550/arXiv.quant-ph/0608197
arXiv:quant-ph/0608197

[4] D. Pérez-García, M. Sanz, C. E. González-Guillén, M. M. Wolf, and J. I. Cirac. “Characterizing symmetries in a projected entangled pair state”. New Journal of Physics 12, 025010 (2010).
https://doi.org/10.1088/1367-2630/12/2/025010

[5] H. Wielandt. “Unzerlegbare, nicht negative Matrizen”. Mathematische Zeitschrift 52, 642–648 (1950).
https://doi.org/10.1007/BF02230720

[6] M. Sanz, D. Pérez-García, M. M. Wolf, and J. I. Cirac. “A quantum version of wielandt’s inequality”. IEEE Transactions on Information Theory 56, 4668–4673 (2010).
https://doi.org/10.1109/TIT.2010.2054552

[7] J. I. Cirac, J. Garre-Rubio, and D. Pérez-García. “Mathematical open problems in projected entangled pair states”. Revista Matemática Complutense 32, 579–599 (2019).
https://doi.org/10.1007/s13163-019-00318-x

[8] A. Molnar, Y. Ge, N. Schuch, and J. I. Cirac. “A generalization of the injectivity condition for projected entangled pair states”. Journal of Mathematical Physics 59, 021902 (2018).
https://doi.org/10.1063/1.5007017

[9] M. Michałek and Y. Shitov. “Quantum version of wielandt’s inequality revisited”. IEEE Transactions on Information Theory 65, 5239–5242 (2019).
https://doi.org/10.1109/TIT.2019.2897772

[10] A. Paz. “An application of the cayley-hamilton theorem to matrix polynomials in several variables”. Linear and Multilinear Algebra 15, 161–170 (1984).
https://doi.org/10.1080/03081088408817585

[11] Y. Shitov. “An improved bound for the lengths of matrix algebras”. Algebra Number Theory 13, 1501–1507 (2019).
https://doi.org/10.2140/ant.2019.13.1501

[12] A. Cadarso, M. Sanz, M. M. Wolf, J. I. Cirac, and D. Pérez-García. “Entanglement, fractional magnetization, and long-range interactions”. Phys. Rev. B 87, 035114 (2013).
https://doi.org/10.1103/PhysRevB.87.035114

[13] V. Lomonosov and P. Rosenthal. “The simplest proof of burnside’s theorem on matrix algebras”. Linear Algebra and its Applications 383, 45–47 (2004).
https://doi.org/10.1016/j.laa.2003.08.012

[14] Y. A. Al’pin and K. D. Ikramov. “Reducibility theorems for pairs of matrices as rational criteria”. Linear Algebra and its Applications 313, 155–161 (2000).
https://doi.org/10.1016/S0024-3795(00)00129-4

[15] Y. A. Al’pin and K. D. Ikramov. “On the unitary similarity of matrix families”. Mathematical Notes 74, 772–782 (2003).
https://doi.org/10.1023/B:MATN.0000009013.89673.0a

[16] W. E. Longstaff and P. Rosenthal. “On the lengths of irreducible pairs of complex matrices”. Proc. Amer. Math. Soc. 139, 3769–3777 (2011).
https://doi.org/10.1090/S0002-9939-2011-11149-3

[17] W. E. Longstaff, A. C. Niemeyer, and O. Panaia. “On the lengths of pairs of complex matrices of size at most five”. Bulletin of the Australian Mathematical Society 73, 461–472 (2006).
https://doi.org/10.1017/S0004972700035462

[18] M. S. Lambrou and W. E. Longstaff. “On the lengths of pairs of complex matrices of size six”. Bulletin of the Australian Mathematical Society 80, 177–201 (2009).
https://doi.org/10.1017/S0004972709000112

[19] C. J. Pappacena. “An upper bound for the length of a finite-dimensional algebra”. Journal of Algebra 197, 535–545 (1997).
https://doi.org/10.1006/jabr.1997.7140

[20] A. Guterman, T. Laffey, O. Markova, and H. Šmigoc. “A resolution of paz’s conjecture in the presence of a nonderogatory matrix”. Linear Algebra and its Applications 543, 234–250 (2018).
https://doi.org/10.1016/j.laa.2018.01.002

[21] M. Rahaman. “A new bound on quantum wielandt inequality”. IEEE Transactions on Information Theory 66, 147–154 (2020).
https://doi.org/10.1109/TIT.2019.2945776

[22] M. B. Hastings. “Random unitaries give quantum expanders”. Phys. Rev. A 76, 032315 (2007).
https://doi.org/10.1103/PhysRevA.76.032315

[23] J. I. Cirac, D. Pérez-García, N. Schuch, and F. Verstraete. “Matrix product states and projected entangled pair states: Concepts, symmetries, theorems”. Rev. Mod. Phys. 93, 045003 (2021).
https://doi.org/10.1103/RevModPhys.93.045003

[24] C. Lancien and D. Pérez-García. “Correlation length in random MPS and PEPS”. Ann. Henri Poincaré 23, 141–222 (2022).
https://doi.org/10.1007/s00023-021-01087-4

[25] D. Perez-Garcia, F. Verstraete, J. I. Cirac, and M. M. Wolf. “Peps as unique ground states of local hamiltonians”. Quant. Inf. Comp. 8, 0650–0663 (2008).
https://doi.org/10.48550/arXiv.0707.2260

[26] M. Michałek, T. Seynnaeve, and F. Verstraete. “A tensor version of the quantum wielandt theorem”. SIAM Journal on Matrix Analysis and Applications 40, 1125–1130 (2019).
https://doi.org/10.1137/18M1227664

[27] N. Schuch, M. M. Wolf, F. Verstraete, and J. I. Cirac. “Computational complexity of projected entangled pair states”. Phys. Rev. Lett. 98, 140506 (2007).
https://doi.org/10.1103/PhysRevLett.98.140506

[28] G. Scarpa, A. Molnár, Y. Ge, J. J. García-Ripoll, N. Schuch, D. Pérez-García, and S. Iblisdir. “Projected entangled pair states: Fundamental analytical and numerical limitations”. Phys. Rev. Lett. 125, 210504 (2020).
https://doi.org/10.1103/PhysRevLett.125.210504

[29] J. Haferkamp, D. Hangleiter, J. Eisert, and M. Gluza. “Contracting projected entangled pair states is average-case hard”. Phys. Rev. Res. 2, 013010 (2020).
https://doi.org/10.1103/PhysRevResearch.2.013010

[30] N. Schuch, M. M. Wolf, F. Verstraete, and J. I. Cirac. “Simulation of quantum many-body systems with strings of operators and monte carlo tensor contractions”. Phys. Rev. Lett. 100, 040501 (2008).
https://doi.org/10.1103/PhysRevLett.100.040501

[31] A. Bonfiglioli and R. Fulci. “Topics in noncommutative algebra: the theorem of Campbell, Baker, Hausdorff and Dynkin”. Volume 2034. Springer Science & Business Media. (2011).
https://doi.org/10.1007/978-3-642-22597-0

[32] J.-P. Serre. “Lie algebras and Lie groups: 1964 lectures given at Harvard University”. Springer. (2009).
https://doi.org/10.1007/978-3-540-70634-2

[33] C. Reutenauer. “Free lie algebras”. In Handbook of Algebra. Volume 3, pages 887–903. North-Holland (2003).
https://doi.org/10.1016/S1570-7954(03)80075-X

[34] S. Lloyd and R. Maity. “Efficient implementation of unitary transformations”. arXiv preprint. (2019). arXiv:1901.03431.
arXiv:1901.03431

[35] D. Elliott. “Bilinear control systems: matrices in action”. Volume 169. Springer Science & Business Media. (2009).
https://doi.org/10.1023/b101451

[36] N. T. Varopoulos, L. Saloff-Coste, and T. Coulhon. “Analysis and geometry on groups”. Cambridge Tracts in Mathematics. Cambridge University Press. (1993).
https://doi.org/10.1017/CBO9780511662485

[37] L. Gao, M. Junge, and N. LaRacuente. “Fisher information and logarithmic Sobolev inequality for matrix-valued functions”. In Annales Henri Poincaré. Volume 21, pages 3409–3478. Springer (2020).
https://doi.org/10.1007/s00023-020-00947-9

[38] W. Arveson. “The probability of entanglement”. Communications in mathematical physics 286, 283–312 (2009).
https://doi.org/10.1007/s00220-008-0661-8

Cited by

[1] Satvik Singh, Mizanur Rahaman, and Nilanjana Datta, “Zero-error communication, scrambling, and ergodicity”, arXiv:2402.18703, (2024).

[2] Jing Bai, Jianquan Wang, and Zhi Yin, “Primitivity for random quantum channels”, Quantum Information Processing 23 2, 47 (2024).

The above citations are from SAO/NASA ADS (last updated successfully 2024-05-03 12:49:23). The list may be incomplete as not all publishers provide suitable and complete citation data.

On Crossref’s cited-by service no data on citing works was found (last attempt 2024-05-03 12:49:21).

This Paper is published in Quantum under the Creative Commons Attribution 4.0 International (CC BY 4.0) license. Copyright remains with the original copyright holders such as the authors or their institutions.